This is the first post in a series on SaaS metrics which cover a variety of SaaS financial metric models using simple mathematical heuristics. In the process, I hope to highlight important relationships between key SaaS metrics and develop a short list of valuable SaaS Metrics Rules-of-Thumb.
SaaS Metrics Rule-of-Thumb #1 – SaaS Churn Kills SaaS Company Growth
Consider a SaaS company that acquires new customers at the constant rate of “b” (for bookings), and has a percentage churn rate of “a” (for attrition). The number of customers after n periods of time “Cn” is given by the following formula:Cn+1 = b + ( 1 – a ) x Cn
Cn = b + b (1-a) + b (1-a)2 + b (1-a)3 … + b(1-a)n-2 + b(1-a)n-1
Cn = b⁄a x ( 1 – ( 1 -a )n )
This formula can be approximated at the two extremes of early growth and maturity.
| Early Growth | Cn = b x n | acquisition rate x time | (n x a << 1) |
| Maturity Limit | Climit = b ÷ a | acquisition rate ÷ % churn rate | (n x a >> 1) |
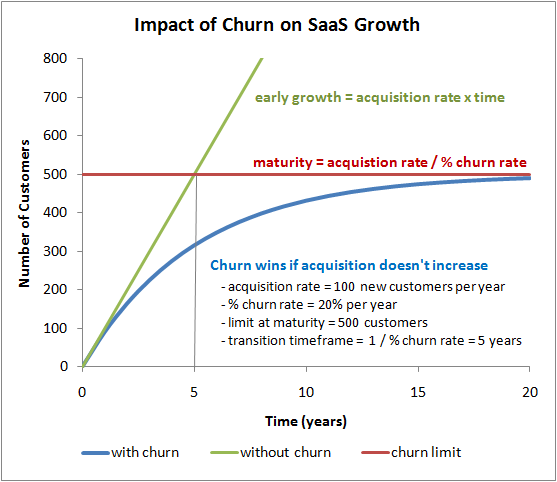
As a SaaS company grows, absolute churn increases with the total number of existing customers and will limit growth if new customers are not added at a faster and faster rate.
SaaS Metrics Rule-of-Thumb #2 – New Customer Acquisition Growth Must Outpace Churn
The bad news is that if you want to grow your SaaS company without limits, you can’t just sit back and book a hundred new customers per year and expect recurring revenue to accumulate, because sooner or later churn catches up with you. You must not only acquire new customers, but you must acquire them at an increasing rate that outpaces your increasing churn.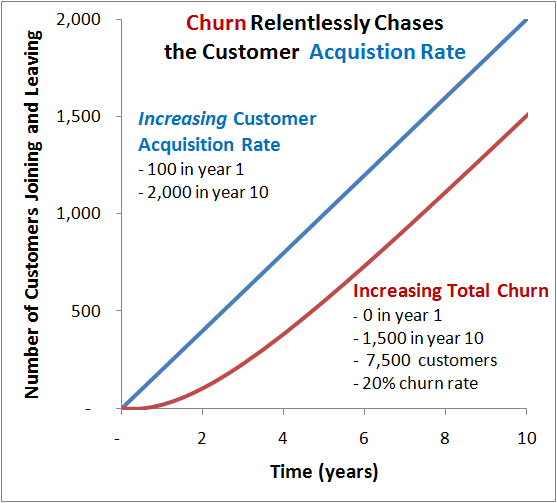
SaaS churn scales with the customer acquisition rate.
In the next post in this SaaS Metrics series, I’ll explore how viral growth is the surest path (albeit not the only path) to achieve the goal of SaaS Metrics Rule-of-Thumb #2 above and break the chains of SaaS churn.
SaaS Metrics Math Notes
This relationship between SaaS churn and SaaS growth can also be derived (somewhat more cleanly) using a continuous model as a function of time, “t”, rather than a discrete model as a function of the number of periods, n. For those that remember their college calculus, the model is represented by the linear first order differential equation: C’(t) = b – a C(t) with the solution: C(t) = b⁄a ( 1 – e-at ). The first graph above is plotted using this continuous solution.
The second SaaS metric rule of thumb above can be shown using asymptotic methods, such that even for increasing customer acquisition b(t), the total SaaS churn rate a C(t) approaches b(t) over time, i.e., SaaS churn chases the acquisition rate.
It is also worth mentioning that this SaaS metrics model applies not only to SaaS, but to any subscription-based business.
This relationship between SaaS churn and SaaS growth can also be derived (somewhat more cleanly) using a continuous model as a function of time, “t”, rather than a discrete model as a function of the number of periods, n. For those that remember their college calculus, the model is represented by the linear first order differential equation: C’(t) = b – a C(t) with the solution: C(t) = b⁄a ( 1 – e-at ). The first graph above is plotted using this continuous solution.
The second SaaS metric rule of thumb above can be shown using asymptotic methods, such that even for increasing customer acquisition b(t), the total SaaS churn rate a C(t) approaches b(t) over time, i.e., SaaS churn chases the acquisition rate.
It is also worth mentioning that this SaaS metrics model applies not only to SaaS, but to any subscription-based business.
